Kirja

One of the key concepts in the impedance analysis is the time constant. As we have seen earlier, a resistor and a capacitor are always paired, and when impedance is determined, the result includes terms with the product RC. The dimension of this product is actually a second, and thus the constant is defined as $\tau$ = RC.  The dimension of the quotient L/R is also a second, but such terms do not exist. We therefore usually say that the circuit in Figure 8.4 is characterized by one time constant, but the circuit in Figure 8.15 has two time constants. The number of the time constants is therefore equal to the number of semicircles in the impedance plot. In the combination of R and C in series, the semicircle is seen in the admittance plot. If the time constants are almost equal in magnitude, it can be difficult to distinguish between them.

In practice, the semicircles observed are rarely perfect, but they seem to be flattened in the imaginary direction. The phenomenon is taken into account by replacing C with a constant phase element (CPE), for which the admittance is

The range of the factor a, i.e. the frequency in Equation (8.88) is in theory [-1,1], but in practice [0,1]. If $\alpha$ = 0, CPE is a resistor and Y₀ = 1/R. When $\alpha$ = 0.5, CPE corresponds to the Warburg element, and $Y_0=\sqrt2/\sigma$ (see (8.22)). If $\alpha$ = 1, CPE is a capacitor and Y₀ = C. If $\alpha$ = -1, CPE is an inductor, and Y₀ = L^-1. The name constant phase element derives from the fact that the angle of the impedance vector is constant independent of frequency:

The flattened semicircle in the impedance plot is obtained when $\alpha$ < 1. Simulated R-CPE circuits with three different values of a are shown in Figure 8.22. The figure illustrates that the smaller $\alpha$, the more the semicircle is flattened.

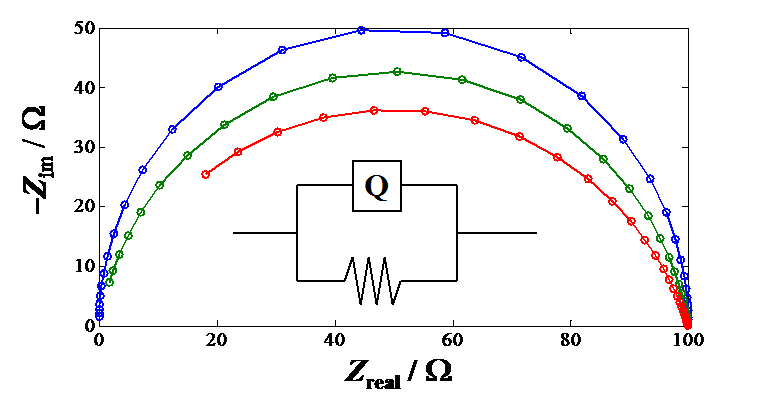
Figure 8.22. Impedance plots of a constant phase element and a resistor in parallel with values of $\alpha$ = 1, 0.9 and 0.8; R = 100 $\Omega$ and Y₀ = 10^-7 $\Omega$^-1. The symbol Q describes the CPE in the fitting software.

In practice, the angle of 45 degrees, which corresponds to the Warburg element, is not reached either, but it remains smaller. In this case, the Warburg element is replaced by CPE, where $\alpha$ < 0.5. Even though it is mathematically possible to take into account the descent of the semicircle and the inclination of the Warburg line using CPE, for every CPE there will be one parameter more in the mathematical treatment. Because of this, it is harder for the iteration to converge, and thus the model may produce erroneous results.

Naturally, the next question we want to ask is about the physical source or interpretation of CPE. The answer lies in the heterogeneity of the electrode surface. First, the surface is far from being completely smooth. Instead, it is rough at least at a molecular level, and usually also at a micrometer level. Second, the electrode surface is not chemically completely heterogeneous either, but it may contain oxidized passive domains or very active areas, for example at the boundaries of crystal surfaces. Because of this, the reaction rate constant and the corresponding charge transfer resistance do not have one single value, but a distribution of values at different points on the surfaces. As a result, the circuit does not have one single time constant, but rather a distribution of time constants. The equivalent circuit of the case is illustrated in Figure 8.23.

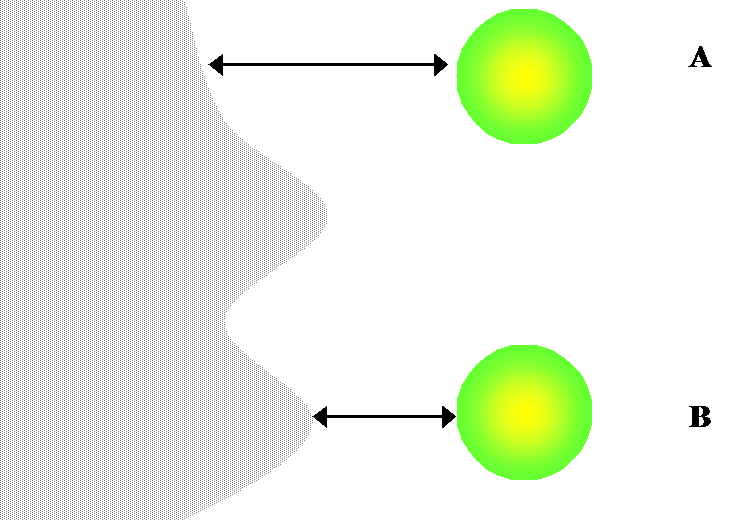
The diffusion element is affected by the surface roughness in a different manner. If the surface of the electrode were completely smooth, the concentration at a given distance from the surface would be constant. But if the surface is rough, there will be equi-concentration planes near the surface of the electrode. Consider Figure 8.24. At a molecular level, diffusion can be seen as Brownian motion. The probability of our test particle hitting the surface of the electrode during a time step Dt is greater at point B than at point A. On the other hand, the average time for the particle to reach the surface is shorter at point B than at point A. The total current observed in experiments therefore provides information on the surface roughness with respect to the average diffusion distance $l \sim\sqrt{D\Delta t}$. This is seen in the potential step experiments (Chapter 7) as the current does not decrease $\sim t^{-1/2}$ but is a bit more gradual, and also the inclination of the Warburg line is lower.