CHEM-E4106 - Electrochemistry D, Lecture, 9.1.2023-21.2.2023

Inserting the dependence of the rate constants on potential it is therefore possible to sketch a current-voltage curve; the only problem comes from the surface concentrations that change as a function of electric current. We begin the analysis with a reversible reaction, i.e. a reaction that has such a high rate that the surface concentrations follow the Nernst equilibrium at all times and in all currents.

6.2.1 Reversible reaction

We consider only the case of a trace ion (see (3.47)) that gives the boundary condition

In a stationary state (steady state) the diffusion equation (3.53) becomes

making concentration profiles linear. As will be explained in Chapter 7, a diffusion boundary layer (DBL) of the thickness $\delta$ is formed in the vicinity of an electrode. With the boundary condition $c_i(\delta)=c_i^b(i=\text{R,O})$ the following result is reached:

In Figure 6.2, steady-state concentration profiles during an oxidation reaction are depicted (anodic current, i > 0).

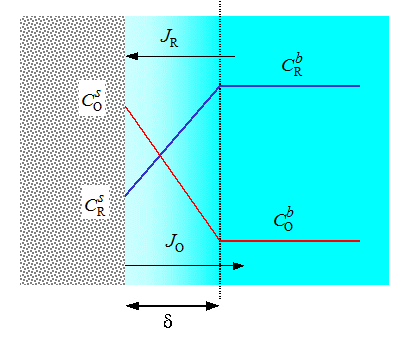
It should be noted that the fluxes of O and R are equal but opposite in direction, J_R = -J_O, in order to maintain the mass balance, as Equation (6.13) actually also shows. As can easily be seen from the picture, (and Equation (6.15) the highest possible current is obtained when the surface concentration of R reaches zero. This current is called the limiting current. Accordingly, if we consider a reduction reaction, the highest possible cathodic current (i < 0) is obtained when the surface concentration of O reaches zero. The anodic and cathodic current densities are respectively obtained from Equation (6.15):

The surface concentrations can be written with the help of current density as:

From Equation (6.16), the bulk concentrations can be solved as a function of the limiting current density, as a result of which the following very useful expressions are obtained:

Equation (6.18) or (6.19) cannot naturally be written for a species that has zero bulk concentration. For example, if $c_{\text{O}}^b=0$, current is always positive and

Figure 6.3 shows current-voltage curves with $i_{\text{lim}}^{red}/{i_{\text{lim}}^{ox}}=0$ or -0.5 and n = 1. The diffusion coefficients are usually rather close to one another, hence $\xi^2 \approx1$. Scaled current density $\chi$ is defined as  $\chi=i/i_{\text{lim}}^{ox}$. Equation (6.21) provides a diagnostic criterion for a reversible electrode reaction: plotting the electrode potential as a function of the logarithm on the right-hand side of Equation (6.21), a straight line is obtained with a slope of 25.7/n mV, or on the log₁₀ scale, 59.1/n mV per decade.

6.2.2 Quasi-reversible reaction

The derived equations above (6.15)-(6.19) are generally valid and can be used in Equation (6.12) to simulate current-voltage curves. Inserting Equation (6.17), for example, we find that

In practice, the curves are preferably presented in terms of measurable quantities. The determination of the thickness of the DBL, $\delta$,  for example, is not unambiguous, as will be seen in Chapter 7.

Let us consider equilibrium again. Now, k_red,eq c_O^b  = k_ox,eq c_R^b  because in the absence of electric current there is no concentration polarization, and surface concentrations are equal to bulk ones. At equilibrium, the rate of oxidation is equal to the rate of the reduction; they are not zero but the equilibrium is a dynamic process where the net reaction rate is zero. The Nernst equation is valid at equilibrium:

Inserting the above equation into the expression of k_red in Equation (6.10), we find that

Exchange current density, i₀, is defined as the inherent rate of oxidation or reduction at equilibrium:

Exchange current density is the measure of the rate of an electrode reaction, i.e. of its reversibility. A good reference electrode, such as Ag/AgCl has an exchange current as high as 10 A/cm². Such an electrode is ideally non-polarizable, as discussed in Chapter 1.

The definition of overpotential is  $\eta=E-E_{eq}$. Hence, $E-E^{0'}=E-E_{eq}+E_{eq}-E^{0'}= \eta+E_{eq}-E^{0'}$. The current-overpotential equation can be derived by applying Equation (6.23) to the last equality:

If the solution is stirred effectively or the current is less than 10% of the limiting current, the concentration polarization can be ignored, and the Butler-Volmer equation is obtained*:

The above equation is traditionally analyzed at limiting values of overpotential. At low overpotentials it can be linearized, giving

The unit of the group of variables RT/(nFi₀) is $\Omega \text{cm}^2$; it is known as the charge transfer resistance, R_ct.

At high positive overpotentials, only the anodic term of Equation (6.28) is significant. Hence

Accordingly, at high negative overpotentials only the cathodic term is significant:

Equations (6.30) and (6.31) are called Tafel equations. The graphs of ln| i | vs. $\eta$ are linear, and their extrapolation to zero overpotential gives the value of i₀. This kind of a graph is called a Tafel plot. The original Tafel equation was empirical and written as

where a and b were experimental constants; b is known as the Tafel slope. Comparing Equations (6.31) and (6.32), the cathodic Tafel slope is†

Very often n = 1 and $\alpha \approx0.5$, giving a typical Tafel slope of approximately 120 mV per current decade. If mass transfer to the electrode is inefficient, the linear range of the plot can be rather limited due to concentration polarization, making the determination of Tafel slopes difficult. Measurements are therefore most often carried out with a rotating disk electrode (see Chapter 7.2.1), which makes the explicit elimination of mass transfer possible.

In a complete current-overpotential curve, mass transfer is taken into account. Inserting Equations (6.19) into Equation (6.27) the following expression can be derived:

$\chi$ is defined similarly to Figure 6.3, $\chi=i/{i_{\text{lim}}^{ox}}$.

Figure 6.4 presents simulated curves using Equation (6.34). It shows the effect of the exchange current density: the lower it is, i.e. the higher $\chi_{\text{kin}}$ is, the wider the potential range where hardly any current flows (potential window). At the limit i₀ → 0 an electrode is ideally polarizable and its i-h graph horizontal. As discussed earlier, an ideally non-polarizable electrode has a very high exchange current density and without mass transfer limitations, its $i-\eta$ graph would be vertical. In Figure 6.4, the curve with $\chi_{\text{kin}}=0.1$ is already completely under mass transfer control because with values lower than that the shape of the curve no longer changes.

An ideally non-polarizable electrode naturally cannot exist because solvent decomposes at high overpotentials. A mercury electrode is closest to an ideally polarizable electrode because the overpotential of hydrogen evolution (see paragraph 6.5.2) on mercury is very high, expanding the potential window over 2 V. Immiscible water-organic interfaces are also polarizable, although the potential window usually remains less than 1 V.

Figure 6.4.  Simulated i-$\eta$ curves with $\chi$_kin = 0.1 -10⁴, $\alpha$ = 0.5, n = 1 or 2 and $\chi$_lim = 2.